Frustrated magnets and fractionalization
Frustrated magnets and fractionalization
One of the attractions of condensed matter physics is how experimental and theoretical developments can go hand-in-hand. In particular, theories can be subjected to experimental tests on reasonable timescales, while experiments frequently throw up puzzles to inspire and challenge theory.
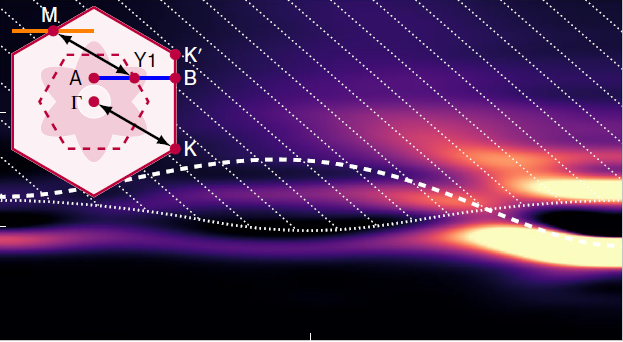
In order to find new types of behaviour, one prerequisite is to find settings where familiar phenomena do not occur. For magnetic system, in order to observe new topological magnetic phases, it is necessary that the conventional types of (anti-)ferromagnetism are sufficiently destabilised to make room for alternative collective instabilities.
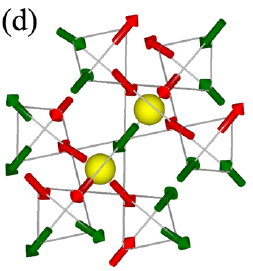
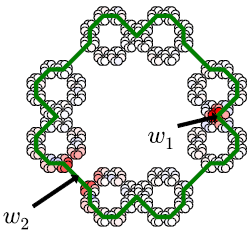
A very productive strategy has been to consider magnets where magnetic interactions compete; in the corresponding models, fundamentally new spin liquid phases with very unusual properties have been generated. These include fractionalised phases which, besides exhibiting topological forms of order, also have exotic quasiparticles, including spinons and holons (which independently carry the magnetic and electric aspects of an electron), Majorana Fermions (which are their own antiparticle) and magnetic monopoles (which carry magnetic charge).
Much of our work, besides developing the theoretical underpinnings of these phenomena, is devoted to understanding, interpreting and guiding experimental efforts to realise such phenomena in materials physics.