
Lévy walks in d dimensions
Lévy walks in d dimensions
Our paper on d-dimensional Lévy walks was published in the Journal of Physics A: Mathematical and Theoretical
Limit theorems for Lévy walks in d dimensions: rare and bulk fluctuations
by Itzhak Fouxon, Sergey Denisov, Vasily Zaburdaev, and Eli Barkai
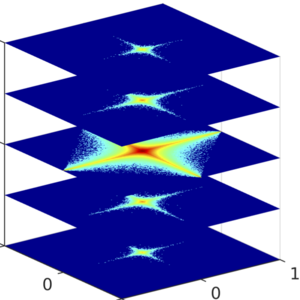
Abstract
We consider super-diffusive Lévy walks in d>=2 dimensions when the duration of a single step, i.e. a ballistic motion performed by a walker, is governed by a power-law tailed distribution of infinite variance and finite mean. We demonstrate that the probability density function (PDF) of the coordinate of the random walker has two different scaling limits at large times. One limit describes the bulk of the PDF. It is the d-dimensional generalization of the one-dimensional Lévy distribution and is the counterpart of the central limit theorem (CLT) for random walks with finite dispersion. In contrast with the one-dimensional Lévy distribution and the CLT this distribution does not have a universal shape. The PDF reflects anisotropy of the single-step statistics however large the time is. The other scaling limit, the so-called ‘infinite density’, describes the tail of the PDF which determines second (dispersion) and higher moments of the PDF. This limit repeats the angular structure of the PDF of velocity in one step. A typical realization of the walk consists of anomalous diffusive motion (described by anisotropic d-dimensional Lévy distribution) interspersed with long ballistic flights (described by infinite density). The long flights are rare but due to them the coordinate increases so much that their contribution determines the dispersion. We illustrate the concept by considering two types of Lévy walks, with isotropic and anisotropic distributions of velocities. Furthermore, we show that for isotropic but otherwise arbitrary velocity distributions the d-dimensional process can be reduced to a one-dimensional Lévy walk. We briefly discuss the consequences of non-universality for the d > 1 dimensional fractional diffusion equation, in particular the non-uniqueness of the fractional Laplacian.
See Publications page for the full