Research
Statistical physics of active agents
Dynamics of biological and synthetic microswimmers
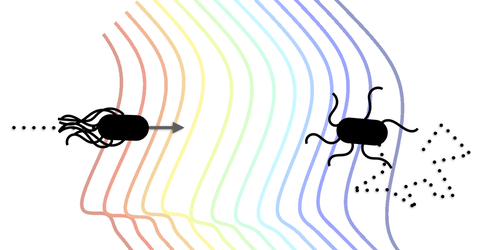
The foundation for investigating, for example, the swimming motion of agents in complex surroundings or their collective behaviors, lies in understanding and quantifying their dynamical behavior at the single-particle level in dilute environments. The spatiotemporal behavior of active (as well as passive or externally driven) particles is encoded in the intermediate scattering function, which constitutes the Fourier transform of the probability density of the particle's displacements. In particular, we have worked on characterizing the dynamical behavior of catalytic Janus colloids using exact solutions for the paradigmatic active Brownian particle model (right, lower panel) and the run-and-tumble behavior of E. coli bacteria in terms of renewal processes. We compare our theoretical predictions to observations of these synthetic and biological micro-swimmers measured by our experimental collaborators.
Relevant publications
Phys. Rev. Lett. 132, 038302 (2024)
Phys. Rev. E 109, 014612 (2024)
Phys. Rev. Lett.121 (2018)
Soft Matter13 (2017)
Sci. Rep.6 (2016)
Press coverage
Newsroom Universität Innsbruck Einfaches Modell für komplexes Phänomen
Physics MagazineCharacterizing the Swimming Gait of a Bacterium
Newsroom Universität InnsbruckBakterien in Bewegung
First-passage-time statistics of active agents
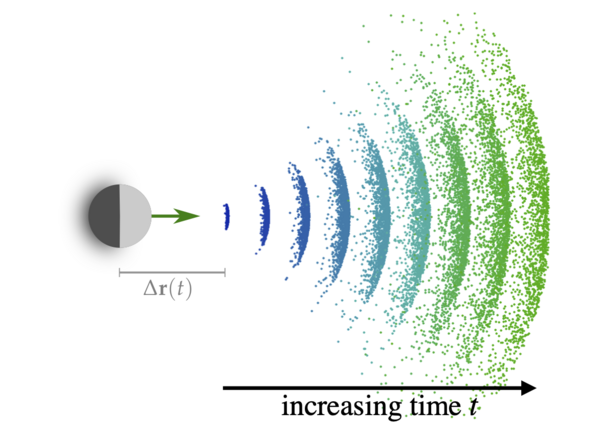
We study the first-passage-time (FPT) properties of active Brownian particles to reach an absorbing wall in two dimensions. Employing a perturbation approach we obtain exact analytical predictions for the survival and FPT distributions for small Péclet numbers, measuring the importance of self-propulsion relative to diffusion. While randomly oriented active agents reach the wall faster than their passive counterpart, their initial orientation plays a crucial role in the FPT statistics. Using the median as a metric, we quantify this anisotropy and find that it becomes more pronounced at distances where persistent active motion starts to dominate diffusion.
Relevant publication
arXiv:2503.05401
Active transport in porous media
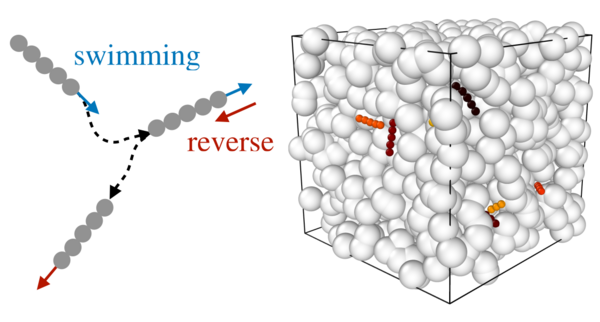
Efficient navigation through disordered, porous environments poses a major challenge for microorganisms and future synthetic cargo-carriers, which hold great potential for novel biomedical and environmental applications. In our work, we model the motion of active agents by self-propelled stiff polymers that employ a run-reverse mechanism at random times. Our findings show that spreading of active agents in porous media can be optimized by tuning their run lengths. We propose a geometric criterion and demonstrate that the optimal spreading occurs when the run lengths are comparable to the longest available pore length of the porous medium. These reversal mechanisms should be included in the strategies for microrobots to traverse porous environments and are relevant for efficient drug-delivery systems and bioremediation tools.
Relevant publication
Press coverage
Princeton press Researchers find the best way for bacteria to navigate maze-like environments
HHU Düsseldorf press Wie Bakterien aus Labyrinthen herausfinden
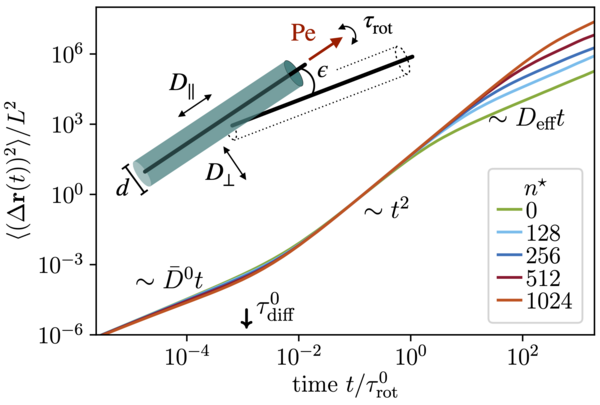
Entangled active matter
`Crowded is slower’ is a well studied problem in many fields ranging from caging in colloidal glasses to the evacuation of crowds to traffic jams. In striking contrast to these observations, we reveal that the diffusivity of self-propelled anisotropic agents, such as biofilaments and elongated bacteria, can be enhanced by orders of magnitude due to crowding. This counterintuitive `crowded is faster’ behavior is corroborated by a scaling theory based on the concept of a confining tube pioneered by Doi and Edwards. Our findings propose a novel way to control the mobility of individual self-propelled anisotropic agents in disordered environments by tuning the degree of crowding.
Relevant publications
arXiv:2310.02929
Phys. Rev. Lett.125 (2020)
Press coverage
Physics Magazine Filaments Move Quickly Through a Crowd
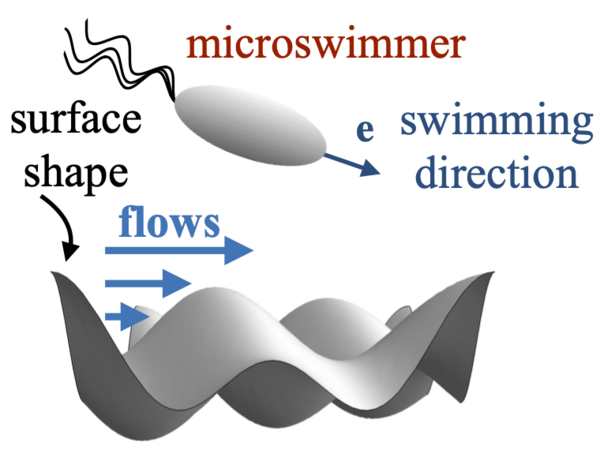
Swimming in confinement: Hydrodynamic couplings
We study hydrodynamic couplings and motion of microorganisms near elastic and corrugated surfaces using a combination of analytical and numerical methods.
Deformable boundaries are omnipresent in the habitats of swimming microorganisms, leading to intricate hydroelastic couplings. Employing a perturbation theory, valid for small deformations, we study the swimming dynamics of pushers and pullers near instantaneously deforming boundaries, endowed with a bending rigidity and surface tension. Our results reveal that pushers can both reorient away from the boundary, leading to overall hydroelastic scattering, or become trapped by the boundary, akin to the enhanced trapping found for pullers. These findings demonstrate that the complex hydroelastic interactions can generate behaviors that are in striking contrast to swimming near planar walls.
Relevant publications
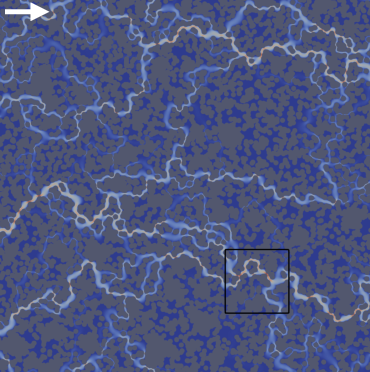
Fluid-structure interactions in microfluidics
Sedimentation and flows near structured surfaces: experiments & theory
Natural and microfluidic environments display a variety of confining surfaces with structured topographies, which modify the surrounding flow fields and and thereby impact the motion of nearby particles via hydrodynamic forces. We study the motion of spherical particles near corrugated surfaces, as are widely used in microfluidic applications, in low-Reynolds-number flows. Comparing quantitatively experiments and theory we show that spheres sedimenting near corrugated surfaces exhibit three-dimensional helical trajectories.
In addition to periodic, wavy surface structures, we study a non-Brownian spherical particle sedimenting nearby a random, rough surface by using an analytical theory and numerical simulations. Roughness of the wall induces fluctuations in the velocity of the fall, leading to a quadratic increase of the variance of the displacements at long times.
Relevant publications
J. Fluid Mech. 982, A31 (2024)
J. Phys.: Cond. Mat. 35, 274003 (2023)
Proc. Natl. Acad. Sci. U.S.A. 119, e2202082119 (2022)
Phys. Rev. Fluids 5, 082101(R) (2020)
Press coverage
Princeton pressA fluid interaction inspired a breakthrough in fluid dynamics
Statistical physics of semiflexible polymers
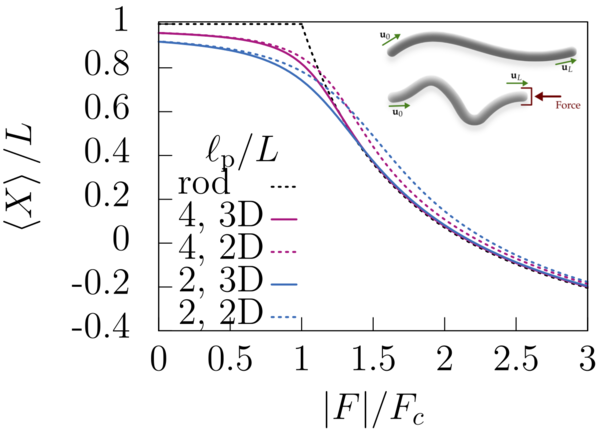
In addition to the dynamics of a self-propelled particle, the shape of its trajectory encodes interesting statistical physics and, in particular, is reminiscent of the contour of a semiflexible polymer, e.g., cytoskeletal filaments. Exploiting this analogy, we calculated analytically the experimentally accessible force-extension relations of semiflexible polymers both in 2D and in 3D, which demonstrates a continuous buckling behavior, in contrast to the Euler buckling instability of a rigid rod. We have also derived analytically the associated probability densities in 2D.
Relvant publications
Soft Matter 14, 7634 (2018)
Soft Matter 14, 2682 (2018)
Phys. Rev. E 95 (2017)
