Research areas

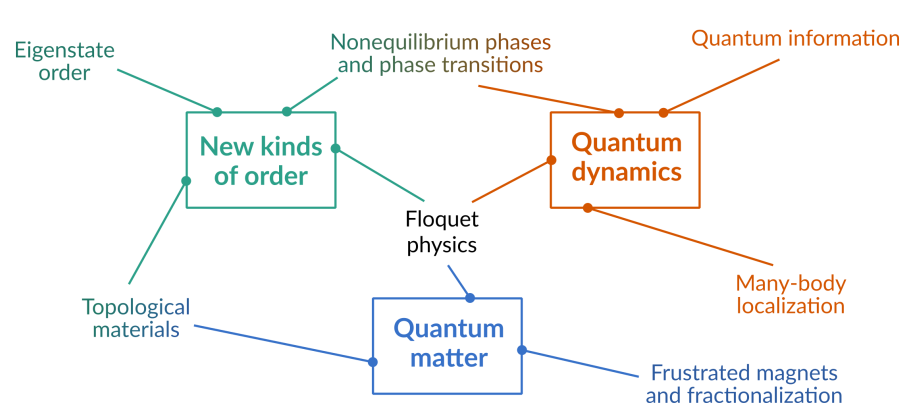
In the Condensed Matter Division we are interested in a broad range of collective phenomena. These can be grouped under three central umbrellas of quantum matter, new kinds of order and quantum dynamics, while still substantial interconnections naturally emerge, see the illustration in the figure above.

A key scope of research is the identification and theoretical description of new kinds of order ranging from unconventional phases such as quantum spin liquids appearing in frustrated magnets to topological matter. These phases often cannot be described by local order parameters but rather entail peculiar entanglement properties linking condensed matter theory directly to quantum information concepts.

The far from equilibrium regime on the other hand, nowadays experimentally accessible in so-called quantum simulators, naturally provides the room for novel quantum states because constraints by equilibrium principles such as the equal a priori probability in the microcanonical ensemble are lifted generically. Prominent examples include the many-body localized phase or eigenstate phases in periodically driven Floquet systems such as discrete time crystals. Detailed descriptions of the individual research topics can be found under the respective embedded links in the figure above.